

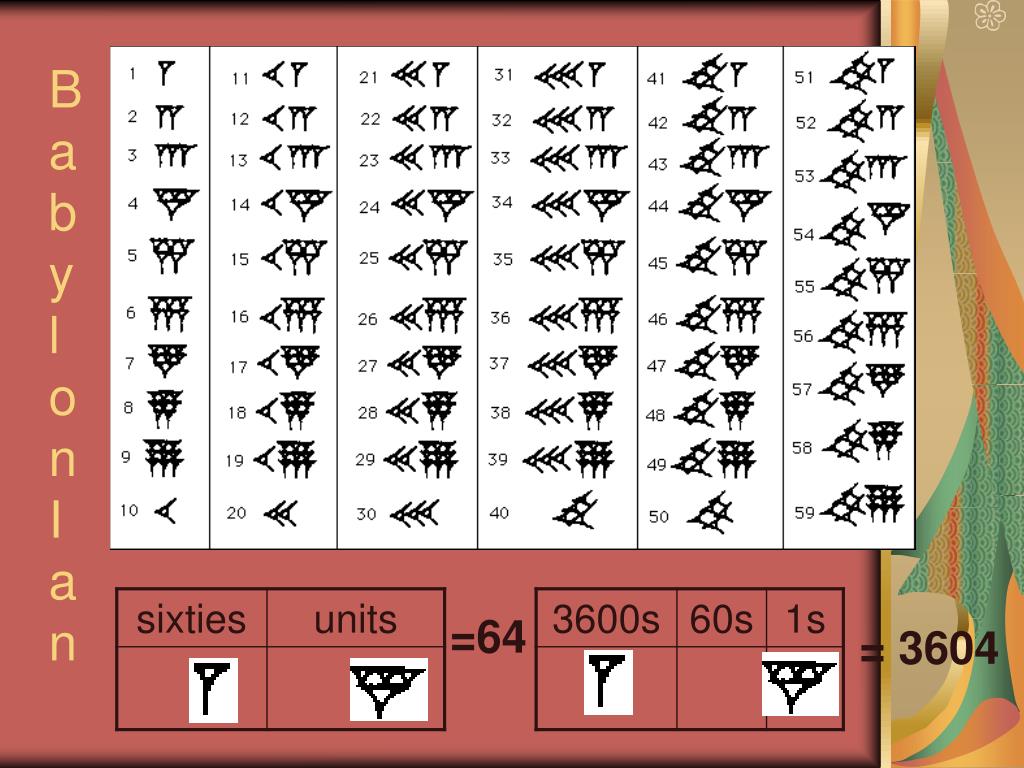
Not only did these two systems use different symbols, they also used different base systems. There were two numeral systems developed by the Mayans-one for the common people and one for the priests. Another important source of information on the Mayans is the writings of Father Diego de Landa, who went to Mexico as a missionary in 1549. In fact, much of what we know about this culture comes from their calendar records and astronomy data. The calendar, and calculations related to it, were thus very important to the ritual life of the priestly class, and hence the Mayan people. This class of priests developed a philosophy with time as divine and eternal. The Mayans had a sophisticated ritual system that was overseen by a priestly class. The Yucatan Peninsula (see figure 16 ) in Mexico was the scene for the development of one of the most advanced civilizations of the ancient world. The Mayan civilization is generally dated from 1500 BCE to 1700 CE. In this chapter, we wrap up with a specific example of a civilization that actually used a base system other than 10. The Babylonians used a base-sixty (sexigesimal) system. For example, the Natives of Queensland used a base-two system, counting as follows: “one, two, two and one, two two’s, much.” Some Modern South American Tribes have a base-five system counting in this way: “one, two, three, four, hand, hand and one, hand and two,” and so on. However, other civilizations have had a variety of bases other than ten. Our own base-ten system probably arose from the fact that we have 10 fingers (including thumbs) on two hands.
Multiplication was carried out using the distributive law. The arithmetic operations are complex in that so many symbols are Mathematics) uses a fractional form identical to ours but with the numerator and denominator in reversed positions. Indeed, Diophantus (who came along very late in Greek Numerous, similar, representations also have been used, with increasing sophistication with time. The numerator was written with an overbar, the denominator with the diacritical mark. More complex fractions could be written as well, with context again being important. A diacritical mark was placed after the denominator of the (unit) fraction. Their notation, however, was ambiguous and context was crucial for the correct reading a fraction. The Greeks used fractions, as did earlier civilizations. The Sand Reckoner where he computed the number of grains of sand to Archimedes extended the system in his book The letter M was used to represent numbers from 10,000 on up.Īlternatively, depending on the history one readsĪs should be evident this system does not allow very large numbers to beĮxpressed. Were represented by placing and apostrophe ' before a unit. Where three additional characters, the (digamma), the However,Ĭalculation lends itself to a great deal of skill within almost any system, the Greek system being no exception.įirst, we note that the number symbols were the same as the letters of the Greek alphabet. This made the system somewhat cumbersome to use.
Adding and subtracting babylonian numerals plus#
Its distinguishing feature is that it was alphabetical and required the use of more than 27 different symbols for numbers plus a couple of other symbols for meaning. Like the Attic and Egyptian systems it was also decimal. The Egyptian though it was non-positional. The (Ionian) Greek system of enumeration was a little more sophisticated than By the Alexandrian Age, the Greek Attic system of enumeration was being replaced by the Ionian or alphabetic numerals. Though there was some steamlining of its use, these symbols were used in a similar way to the Egyptian system, being that symbols were used repeatedly as needed and the system was non positional.

The earliest numerical notation used by the Greeks was the Attic system.


 0 kommentar(er)
0 kommentar(er)
